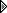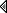[jrblasde]

I have reattempted with short leads to the inductors and with some resistance inserted on the primary side (I started with a 15 kΩ as the output resistance of the 14F8 on the primary side is 14.5 kΩ, but lowered it down to 4.7 kΩ for better readings). The results were more stable (with both the individual inductors and with the coupled inductors), but still I felt that the individual inductors worked better. I am still noticing that there’s no peaking or attenuation as I adjust the trim capacitors, so I believe that I’m still facing added parasitic capacitance from something.

My next plan of attack is to reduce the inductance while increasing the capacitance. I should be able to use a 3.3 μH inductor with a total of 92.69 pF in capacitance. I could use an 82 pF fixed capacitor and a 6.8pF fixed capacitor in parallel with each other for a fixed capacitance of 88.8 pF, in parallel with a 10 pF trim capacitor. This should raise the capacitance enough that any minor interference will not drastically affect the resonant frequency.

I thought about determining the theoretical inductance of the existing, broken IF transformer’s coils. There are 15 turns at a diameter of 0.175” and a solenoid length of 0.15”. The formula for inductance of a coil is L = (N^2 * μ * Α) / l, where N is the number of turns, μ is the permeability, A is the cross sectional area, and L is the length of the coil. I calculate 4.61 μH for the inductance with no iron core. I should be closer to this value if I use the 3.3 μH inductors.

Joseph